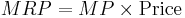Marginal revenue productivity theory of wages
The marginal revenue productivity theory of wages, also referred to as the marginal revenue product of labor and the value of the marginal product or VMPL, is the change in total revenue earned by a firm that results from employing one more unit of labor. It is a neoclassical model that determines, under some conditions, the optimal number of workers to employ at an exogenously determined market wage rate.[1]
The idea that payments to factors of production equilibrate to their marginal productivity had been laid out early on by such as John Bates Clark and Knut Wicksell, who presented a far simpler and more robust demonstration of the principle. Much of the present conception of that theory stems from Wicksell's model.
The marginal revenue product (MRP) of a worker is equal to the product of the marginal product of labor (MP) and the marginal revenue (MR), given by MR×MP = MRP. The theory states that workers will be hired up to the point where the Marginal Revenue Product is equal to the wage rate by a maximizing firm, because it is not efficient for a firm to pay its workers more than it will earn in profits from their labor.
Contents |
Mathematical Relation
The marginal revenue product of labour MRPL is the increase in revenue per unit increase in the variable input = ∆TR/∆L
MR = ∆TR/∆Q
MPL = ∆Q/∆L
MR x MPL = (∆TR/∆Q) x (∆Q/∆L) = ∆TR/∆L
Note that the change in output is not limited to that directly attributable to the additional worker. Assuming that the firm is operating with diminishing marginal returns then the addition of an extra worker reduces the average productivity of every other worker (and every other worker affects the marginal productivity of the additional worker) - in English everybody is getting in each other's way.
As above noted the firm will continue to add units of labor until the MRPL = w
Mathematically until
MRPL = w
MR(MPL) = w
MR = w/MPL
MR = MC which is the profit maximizing rule.
Marginal Revenue Product in a perfectly competitive market
Under perfect competition, marginal revenue product is equal to marginal physical product (extra unit produced as a result of a new employment) multiplied by price.
This is because the firm in perfect competition is a price taker. It does not have to lower the price in order to sell additional units of the good.
MRP in monopoly or imperfect competition
Firms operating under conditions of monopoly or imperfect competition are faced with downward sloping demand curves. If they want to sell extra units of output, they must lower price. Under such market conditions, marginal revenue product will not equal MPP×Price. This is because the firm is not able to sell output at a fixed price per unit.
The MRP curve of a firm in monopoly or imperfect competition will slope downwards at a faster rate than in perfect competition. This can be explained as follows:
- MPP slopes downwards because of the operation of the Law of Diminishing Returns. MRP depends on MPP.
- Because the firm faces a downward sloping demand curve for its product, it must lower price to sell extra units of output. Yh
References
- ^ See Daniel S. Hamermesh, "The demand for labor in the long run"; published in Handbook of Labor Economics (Orley Ashenfelter and Richard Layard, ed.), 1986, p. 429.